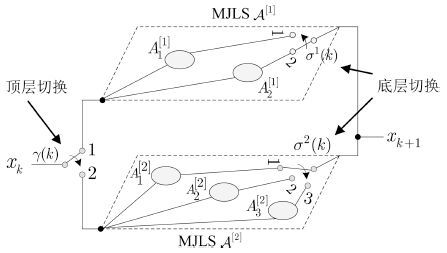
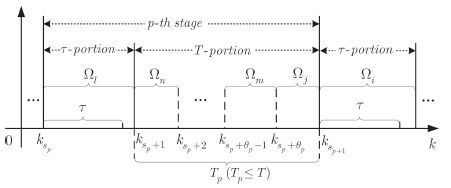
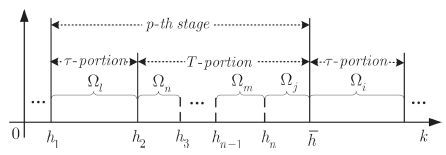
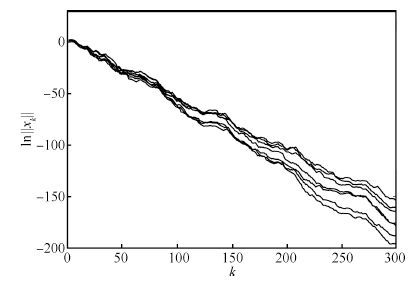
|
[1]
|
Briat C, Seuret A. Affine characterizations of minimal and mode-dependent dwell-time for uncertain switched linear systems. IEEE Transactions on Automatic Control, 2013, 58(5):1304-1310 doi: 10.1109/TAC.2012.2220031
|
|
[2]
|
Zhao X D, Zhang L X, Shi P, Liu M. Stability and stabilization of switched linear systems with mode-dependent average dwell time. IEEE Transactions on Automatic Control, 2012, 57(2):1809-1815 http://ieeexplore.ieee.org/document/6097035/
|
|
[3]
|
Hespanha J P. Uniform stability of switched linear systems:extensions of LaSalle's invariance principle. IEEE Transactions on Automatic Control, 2004, 49(4):470-482 doi: 10.1109/TAC.2004.825641
|
|
[4]
|
Zhang L X, Zhuang S L, Shi P. Non-weighted quasi-time-dependent H∞ filtering for switched linear systems with persistent dwell-time. Automatica, 2015, 54:201-209 doi: 10.1016/j.automatica.2015.02.010
|
|
[5]
|
Zhu J, Wang L P, Spiryagin M. Control and decision strategy for a class of Markovian jump systems in failure prone manufacturing process. IET Control Theory & Applications, 2012, 6(12):1803-1811
|
|
[6]
|
Mao Z, Jiang B, Shi P. H∞ fault detection filter design for networked control systems modelled by discrete Markovian jump systems. IET Control Theory & Applications, 2007, 1(5):1336-1343 http://ieeexplore.ieee.org/document/4293139/keywords
|
|
[7]
|
马卫国, 邵诚. 网络控制系统随机稳定性研究. 自动化学报, 2007, 33(8):878-882 http://www.aas.net.cn/CN/abstract/abstract17261.shtmlMa Wei-Guo, Shao Cheng. Stochastic stability for networked control systems. Acta Automatica Sinica, 2007, 33(8):878-882 http://www.aas.net.cn/CN/abstract/abstract17261.shtml
|
|
[8]
|
Xiao N, Xie L H, Fu M Y. Stabilization of Markov jump linear systems using quantized state feedback. Automatica 2010, 46(10):1696-1702 doi: 10.1016/j.automatica.2010.06.018
|
|
[9]
|
Bolzern P, Colaneri P, De Nicolao G. Stochastic stability of positive Markov jump linear systems. Automatica 2014, 50(4):1181-1187 doi: 10.1016/j.automatica.2014.02.016
|
|
[10]
|
Fang Y G, Loparo K A. Stabilization of continuous-time jump linear systems. IEEE Transactions on Automatic Control, 2002, 47(10):1590-1603 doi: 10.1109/TAC.2002.803528
|
|
[11]
|
Song Y, Dong H, Yang T C, Fei M R. Almost sure stability of discrete-time Markov jump linear systems. IET Control Theory & Applications, 2014, 8(11):901-906
|
|
[12]
|
Xiong J L, Lam J, Shu Z, Mao X R. Stability analysis of continuous-time switched systems with a random switching signal. IEEE Transactions on Automatic Control, 2014, 59(1):180-186 doi: 10.1109/TAC.2013.2266751
|
|
[13]
|
Bolzern P, Colaneri P, De Nicolao G. Almost sure stability of Markov jump linear systems with deterministic switching. IEEE Transactions on Automatic Control, 2013, 58(1):209-214 doi: 10.1109/TAC.2012.2203049
|
|
[14]
|
Bolzern P, Colaneri P, De Nicolao G. Markov jump linear systems with switching transition rates:mean square stability with dwell-time. Automatica, 2010, 46(6):1081-1088 doi: 10.1016/j.automatica.2010.03.007
|
|
[15]
|
盖彦荣, 陈阳舟, 张亚霄. 切换信息拓扑和时变时滞下离散时间线性多智能体系统一致性的平均驻留时间条件. 自动化学报, 2014, 40(11):2609-2617 doi: 10.1016/S1874-1029(14)60407-9Ge Yan-Rong, Chen Yang-Zhou, Zhang Ya-Xiao. Average dwell-time conditions for consensus of discrete-time linear multi-agent systems with switching topologies and time-varying delays. Acta Automatica Sinica, 2014, 40(11):2609-2617 doi: 10.1016/S1874-1029(14)60407-9
|
|
[16]
|
Tanelli M, Picasso B, Bolzern P, Colaneri P. Almost sure stabilization of uncertain continuous-time Markov jump linear systems. IEEE Transactions on Automatic Control, 2010, 55(1):195-201 doi: 10.1109/TAC.2009.2033844
|
|
[17]
|
Bolzern P, Colaneri P, De Nicolao G. On almost sure stability of continuous-time Markov jump linear systems. Automatica, 2006, 42(6):983-988 doi: 10.1016/j.automatica.2006.02.007
|
|
[18]
|
Resnick S I. Adventures in Stochastic Processes. Basel, Switzerland:Birkhäuser Verlag, 1992.
|




 下载:
下载: