|
[1]
|
He S D, and Wang M, and Dai S L, and Luo F. Leader-follower formation control of USVs with prescribed performance and collision avoidance. IEEE Transactions on Industrial Informatics, 2019, 15(1): 572−581 doi: 10.1109/TII.2018.2839739
|
|
[2]
|
Li Y M, and Feng K L, and Li K W. Finite-time fuzzy adaptive dynamic event-triggered formation tracking control for USVs with actuator faults and multiple constraints. IEEE Transactions on Industrial Informatics, 2024, 20(4): 5285−5296 doi: 10.1109/TII.2023.3331101
|
|
[3]
|
Olfati-Saber R, Fax J A, Murray R M. Consensus and cooperation in networked multi-agent systems. Proceedings of the IEEE, 2007, 95(1): 215−233 doi: 10.1109/JPROC.2006.887293
|
|
[4]
|
Guo X Y, and Zhang H G, and Sun J Y, Zhou Y. Fixed-time fuzzy adaptive control of manipulator systems under multiple constraints: A modified dynamic surface control approach. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2023, 53(4): 2522−2532 doi: 10.1109/TSMC.2022.3212988
|
|
[5]
|
Ren H R, Cheng Z J, Qin J H, Lu R Q. Deception attacks on event-triggered distributed consensus estimation for nonlinear systems. Automatica, 2023, 154: 111100 doi: 10.1016/j.automatica.2023.111100
|
|
[6]
|
Wu Y, Chen M, Chadli M, Li H Y. Dual-type-triggers-based cooperative adaptive critic control of swarm UAVs under FDI attacks. Automatica, 2024, 167: 111757 doi: 10.1016/j.automatica.2024.111757
|
|
[7]
|
应晨铎, 伍益明, 徐明, 郑宁, 何熊熊. 欺骗攻击下具备隐私保护的多智能体系统均值趋同控制. 自动化学报, 2023, 49(2): 425−436Ying C D, Wu Yi M, Xu M, Zheng N, He X X. Privacy-preserving average consensus control formulti-agent systems under deception attacks. Acta Automatica Sinica, 2023, 49(2): 425−436
|
|
[8]
|
杨彬, 周琪, 曹亮, 鲁仁全. 具有指定性能和全状态约束的多智能体系统事件触发控制. 自动化学报, 2019, 45(8): 1527−1535Yang B, Zhou Q, Cao L, Lu R Q. Event-triggered control for multi-agent systems with prescribed performance and full state constraints. Acta Automatica Sinica, 2019, 45(8): 1527−1535
|
|
[9]
|
Li H Q, Liao X F, Huang T W, Zhu W. Event-triggering sampling based leader-following consensus in second-order multi-agent systems. IEEE Transactions on Automatic Control, 2015, 60(7): 1998−2003 doi: 10.1109/TAC.2014.2365073
|
|
[10]
|
Li K, Hua C C, You X, Ahn C K. Leader-following consensus control for uncertain feedforward stochastic nonlinear multiagent systems. IEEE Transactions on Neural Networks and Learning Systems, 2023, 34(2): 1049−1057 doi: 10.1109/TNNLS.2021.3105109
|
|
[11]
|
Yao D Y, Li H Y, Shi Y. Event-based average consensus of disturbed MASs via fully distributed sliding mode control. IEEE Transactions on Automatic Control, 2024, 69(3): 2015−2022 doi: 10.1109/TAC.2023.3317505
|
|
[12]
|
Li X J, Bo S, Zhang X W, Qin Y, Yin X Y. Data-driven parallel koopman subsystem modeling and distributed moving horizon state estimation for large-scale nonlinear processes. AIChE Journal, 2024, 70(3): e18326 doi: 10.1002/aic.18326
|
|
[13]
|
范泉涌, 张乃宗, 唐勇, 许斌. 基于动态事件触发通信协议的多智能体系统自适应可靠控制. 自动化学报, 2024, 50(5): 924−936Fan Q Y, Zhang N Z, Tang Y, Xu B. Adaptive reliable control of multi-agent systems based on dynamic event-triggered communication protocol. Acta Automatica Sinica, 2024, 50(5): 924−936
|
|
[14]
|
Mei J, Ren W, Song Y D. A unified framework for adaptive leaderless consensus of uncertain multiagent systems under directed graphs. IEEE Transactions on Automatic Control, 2021, 66(12): 6179−6186 doi: 10.1109/TAC.2021.3062594
|
|
[15]
|
Ma D, Chen J Q, Lu R Q, Chen J, Chai T Y. Delay consensus margin of first-order multiagent systems with undirected graphs and pd protocols. IEEE Transactions on Automatic Control, 2021, 66(9): 4192−4198 doi: 10.1109/TAC.2020.3035556
|
|
[16]
|
Psillakis H E. Consensus in networks of agents with unknown high-frequency gain signs and switching topology. IEEE Transactions on Automatic Control, 2017, 62(8): 3993−3998 doi: 10.1109/TAC.2016.2616645
|
|
[17]
|
Wen G H, Zheng W X. On constructing multiple lyapunov functions for tracking control of multiple agents with switching topologies. IEEE Transactions on Automatic Control, 2019, 64(9): 3796−3803 doi: 10.1109/TAC.2018.2885079
|
|
[18]
|
孙梦薇, 任璐, 刘剑, 孙长银. 切换拓扑下动态事件触发多智能体系统固定时间一致性. 自动化学报, 2023, 49(6): 1295−1305Sun M W, Ren L, Liu J, Sun C Y. Dynamic event-triggered fixed-time consensus control of multi-agent systems under switching topologies. Acta Automatica Sinica, 2023, 49(6): 1295−1305
|
|
[19]
|
Olfati-Saber R, Murray R M. Consensus problems in networks of agents with switching topology and time-delays. IEEE Transactions on Automatic Control, 2004, 49(9): 1520−1533 doi: 10.1109/TAC.2004.834113
|
|
[20]
|
Wang P J, Wen G H, Yu W W, Huang T W, Yu X H. Asymptotical consensus of mimo linear multiagent systems with a nonautonomous leader and directed switching topology: a continuous approach. IEEE Transactions on Control of Network Systems, 2023, 10(3): 1326−1337 doi: 10.1109/TCNS.2022.3226944
|
|
[21]
|
Razaq, M A, Rehan M, Tufail M, Ahn C K. Multiple lyapunov functions approach for consensus of one-sided lipschitz multi-agents over switching topologies and input saturation. IEEE Transactions on Circuits and Systems Ⅱ: Express Briefs, 2020, 67(12): 3267−3271 doi: 10.1109/TCSII.2020.2986009
|
|
[22]
|
Frasca P, and Carli R, and Fagnani F, and Zampieri S. Average consensus on networks with quantized communication. International Journal of Robust and Nonlinear Control: IFAC-Affiliated Journal, 2009, 19(16): 1787−1816
|
|
[23]
|
Liu S, and Li T, and Xie L H, and Fu M Y, and Zhang J-F. Continuous-time and sampled-data-based average consensus with logarithmic quantizers. Automatica, 2013, 49(11): 3329−3336 doi: 10.1016/j.automatica.2013.07.016
|
|
[24]
|
Hu M, Wang T, Zhao Y L. Consensus of switched multi-agent systems with binary-valued communications. Science China Information Sciences, 2022, 65(6): 162207 doi: 10.1007/s11432-020-3052-0
|
|
[25]
|
Ning Z P, Feng G, Yin X Y. Asynchronous quantized control of piecewise-affine systems. IEEE Transactions on Automatic Control, 2024, 69(1): 503−510 doi: 10.1109/TAC.2023.3266978
|
|
[26]
|
Wang L Y, Zhang J F, Yin G G. System identification using binary sensors. IEEE Transactions on Automatic Control, 2003, 48(11): 1892−1907 doi: 10.1109/TAC.2003.819073
|
|
[27]
|
Zhao Y L, Wang T, Bi W J. Consensus protocol for multiagent systems with undirected topologies and binary-valued communications. IEEE Transactions on Automatic Control, 2019, 64(1): 206−221 doi: 10.1109/TAC.2018.2814632
|
|
[28]
|
Wang T, Zhang H, Zhao Y L. Consensus of multi-agent systems under binary-valued measurements and recursive projection algorithm. IEEE Transactions on Automatic Control, 2020, 65(6): 2678−2685 doi: 10.1109/TAC.2019.2942569
|
|
[29]
|
Chen J N, Hua C C. Adaptive iterative learning fault-tolerant consensus control of multiagent systems under binary-valued communications. IEEE Transactions on Cybernetics, 2023, 53(11): 6751−6760 doi: 10.1109/TCYB.2021.3123697
|
|
[30]
|
Li X J, Bo S, Qin Y, Yin X Y. Iterative distributed moving horizon estimation of linear systems with penalties on both system disturbances and noise. Chemical Engineering Research and Design, 2023, 194: 878−893 doi: 10.1016/j.cherd.2023.05.020
|
|
[31]
|
Ahn H S, Chen Y Q, Moore K L. Iterative learning control: Brief survey and categorization. IEEE Transactions on Systems, Man, and Cybernetics, Part C (Applications and Reviews), 2007, 37(6): 1099−1121 doi: 10.1109/TSMCC.2007.905759
|
|
[32]
|
Yang S P, Xu J X, Li X F, Shen D. Iterative learning control for multiagent systems coordination. John Wiley & Sons, 2017.
|
|
[33]
|
Bu X H, Yu Q X, Hou Z S, and Qian W. Model free adaptive iterative learning consensus tracking control for a class of nonlinear multiagent systems. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2019, 49(4): 677−686 doi: 10.1109/TSMC.2017.2734799
|
|
[34]
|
Ren H R, Ma H, Li H Y, Wang Z Y. Adaptive fixed-time control of nonlinear MASs with actuator faults. IEEE/CAA Journal of Automatica Sinica, 2023, 10(5): 1252−1262 doi: 10.1109/JAS.2023.123558
|
|
[35]
|
Li K, Ding S X, Zheng W X, Hua C C. Global distributed fault-tolerant consensus control of nonlinear delayed multiagent systems with hybrid faults. IEEE Transactions on Automatic Control, 2024, 69(3): 1967−1974 doi: 10.1109/TAC.2023.3303098
|
|
[36]
|
Cai Y L, Zhang H G, Li W H, Mu Y F, He Q. Distributed bipartite adaptive event-triggered fault-tolerant consensus tracking for linear multiagent systems under actuator faults. IEEE Transactions on Cybernetics, 2022, 52(11): 11313−11324 doi: 10.1109/TCYB.2021.3069955
|
|
[37]
|
Wang C L, Wen C Y, Guo L. Adaptive consensus control for nonlinear multiagent systems with unknown control directions and time-varying actuator faults. IEEE Transactions on Automatic Control, 2021, 66(9): 4222−4229 doi: 10.1109/TAC.2020.3034209
|
|
[38]
|
Chen S, Ho D W C, Li L L, Liu M. Fault-tolerant consensus of multi-agent system with distributed adaptive protocol. IEEE Transactions on Cybernetics, 2015, 45(10): 2142−2155 doi: 10.1109/TCYB.2014.2366204
|
|
[39]
|
Li H Y, Wu Y, Chen M. Adaptive fault-tolerant tracking control for discrete-time multiagent systems via reinforcement learning algorithm. IEEE Transactions on Cybernetics, 2021, 51(3): 1163−1174 doi: 10.1109/TCYB.2020.2982168
|



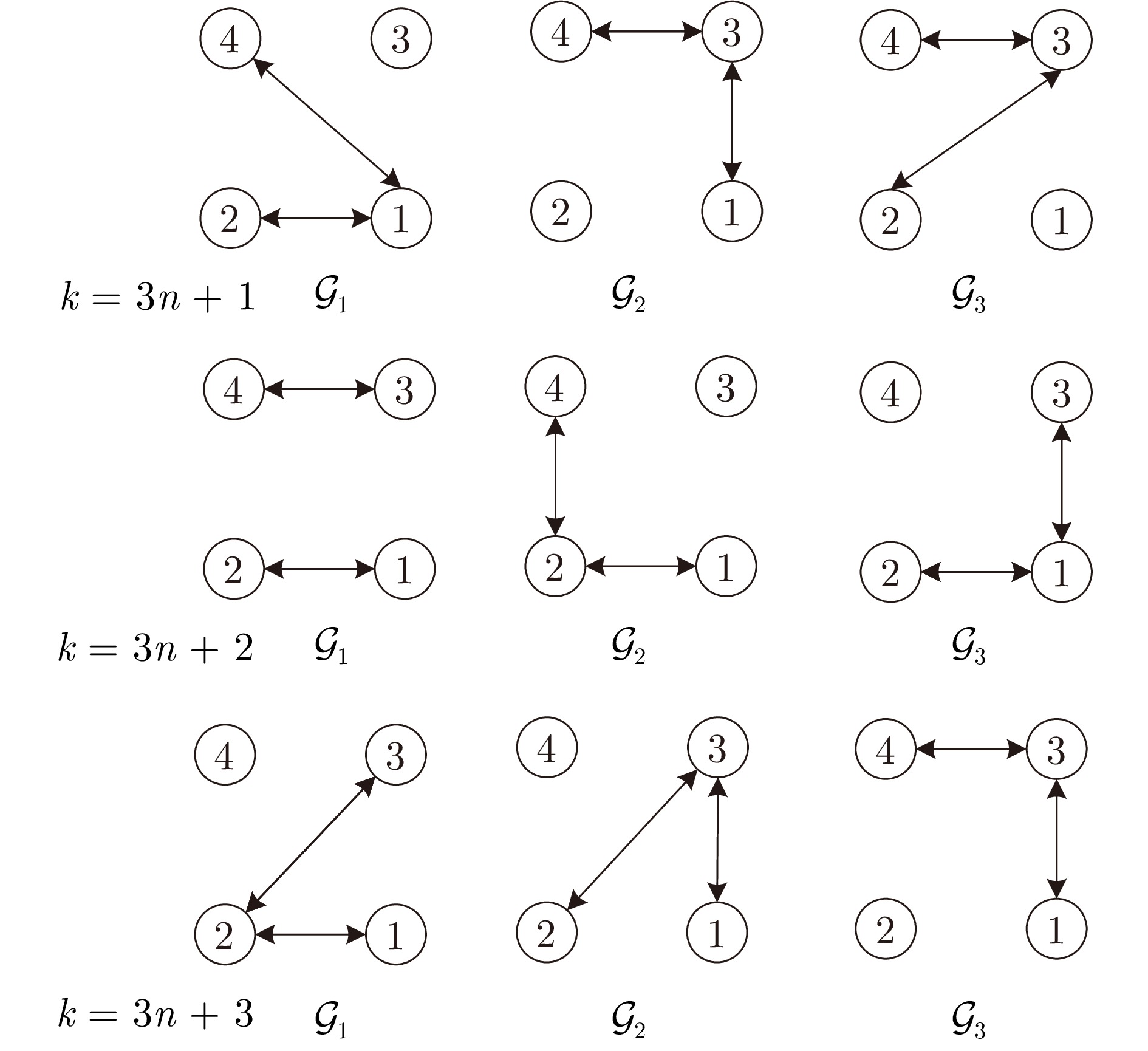
 下载:
下载: