Sequential Equilibrium Analysis and Computational Experiments of a Bargaining Game with Incomplete Information
-
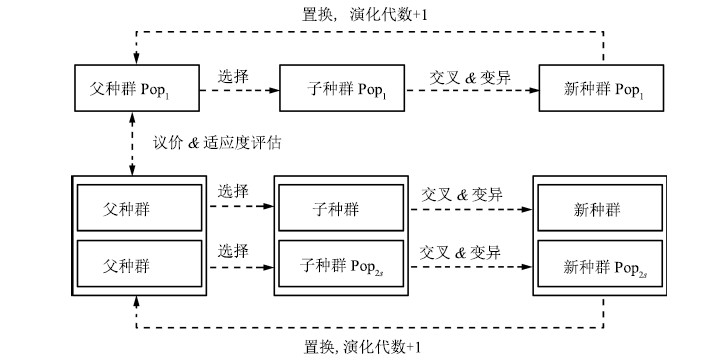
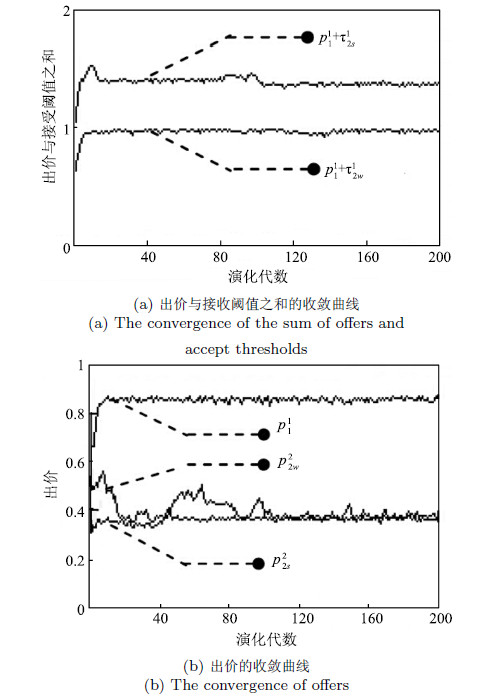
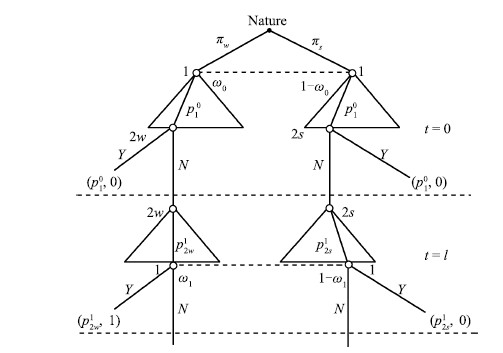
摘要: 本文从理论研究和计算实验两个层次分析和验证了一类带有时间 偏好的单边双类型不完全信息议价博弈模型及其序贯均衡, 运用单阶段偏离法则分别推导和证明了该议价博弈的合并均衡与分离均衡, 并通过策略比较和构造静态出价博弈证明了合并均衡是议价博弈的唯一理性解. 在此基础上, 本文设计不完全信息议价博弈计算实验场景, 基于协同演化计算实验方法验证了议价博弈的序贯均衡解. 最后, 本文探讨了该序贯均衡对于议价双方相应管理策略的实践指导意义.Abstract: This paper analyzes and experimentally validates the sequential equilibrium of a bargaining game with one-sided incomplete information about players' time preferences. Using one-stage-deviation principle, we deduce the pooling equilibrium and separating equilibrium of this bargaining game, respectively, and we prove by strategy comparison and constructing a static offer game that the pooling equilibrium is the unique rational solution to the bargaining model. We also design the computational experiments for the bargaining game with incomplete information, and quantitively validate the sequential equilibrium solution via co-evolution-based computational experiments. Towards the end, we discuss the practical significance of our research findings.
-
表 1 静态出价博弈的支付矩阵
Table 1 The payoff matrix of the static offer game
2s 2w $p_{2s}^0 = {{\hat V}_s}$ $p_{2s}^0=y^{\omega_0}$ $p_{2w}^0 = {{\hat V}_w}$ $\;({{\hat V}_w},0)({y^{{\omega _0}}},2)$ $({{\hat V}_w},0)({y^{{\omega _0}}},0)$ $p_{2w}^0 = P_{2s}^0$ $({x^{{\omega _0}}},1)({y^{{\omega _0}}},2)$ $({y^{{\omega _0}}},0)({y^{{\omega _0}}},0)$ 表 2 计算实验场景的参数设置
Table 2 The parameters of the computational experiments
贴现因子 初始信念 议价期数 δ1= 0.6, δw= 0.2, δw=0.8 ω= 0.6 T = 100 -
[1] Rubinstein A. Perfect equilibrium in a bargaining model. Econometrica, 1982, 50(1): 97-109 [2] Rubinstein A. A bargaining model with incomplete information about time preferences. Econometrica, 1985, 53(5): 1151-1172 [3] 李军林, 李天有. 讨价还价理论及其最近的发展. 经济理论与经济管理, 2005, (3): 63-67Li Jun-Lin, Li Tian-You. Bargaining theory and the recent development. Economic Theory and Business Management, 2005, (3): 63-67 [4] Cramton P C. Strategic delay in bargaining with two-sided uncertainty. The Review of Economic Studies, 1992, 59(1): 205-225 [5] Schweinzer P. Sequential bargaining with common values. Journal of Mathematical Economics, 2010, 46(1): 109-121 [6] Li D Z. Multiplicity of equilibrium payoffs in three-player baron-ferejohn model. Economics Bulletin, 2014, 34(2): 1122-1132 [7] 王刊良, 王嵩. 非对称信息下讨价还价的动态博弈: 以三阶段讨价还价为例. 系统工程理论与实践, 2010, 30(9): 1636-1642Wang Kan-Liang, Wang Song. Dynamic game of asymmetry information bargaining with tri-stages bargaining as example. Systems Engineering-Theory and Practice, 2010, 30(9): 1636-1642 [8] Karni E, Shmuel Z. A model of bargaining with incomplete information [Online], available: http://www.econ.hku.hk/ workshop/2007/Kz101007.pdf, September 1, 2015 [9] Akin Z. Imperfect information processing in sequential bargaining games with present biased preferences. Journal of Economic Psychology, 2009, 30(4): 642-650 [10] Bo A, Gatti N, Lesser V. Bilateral bargaining with one-sided two-type uncertainty. In: Proceedings of the 2009 IEEE/WIC/ACM International Joint Conferences on Web Intelligence and Intelligent Agent Technology. Milan, Italy: IET, 2009. 403-410 [11] Sánchez-Anguix V, Valero S, Julián V, Botti V, García-Fornes A. Genetic-aided multi-issue bilateral bargaining for complex utility functions. In: Proceedings of the 9th International Conference on Autonomous Agents and Multiagent Systems. Toronto, Canada, 2010. 1601-1602 [12] Fatima S S, Wooldridge M, Jennings N R. Bargaining with incomplete information. Annals of Mathematics and Artificial Intelligence, 2005, 44(3): 207-232 [13] 杜义飞, 李仕明, 林光平. 讨价还价过程与供应链的利润最大化均衡. 中国管理科学, 2006, 14(1): 37-42Du Yi-Fei, Li Shi-Ming, Lin Guang-Ping. Bargaining process and equilibrium of profit-maximizing for supply chain. Chinese Journal of Management Science, 2006, 14(1): 37-42 [14] 向钢华, 王永县. 一种不完全信息相互威慑讨价还价模型. 运筹与管理, 2008, 17(6): 16-19Xiang Gang-Hua, Wang Yong-Xian. A bargaining model of mutual deterrence with incomplete information. Operations Research and Management Science, 2008, 17(6): 16-19 [15] Osborne M J, Rubinstein A. Bargaining and Markets. San Diego, California: Academic Press Inc., 1990. [16] Rubinstein A. Choice of conjectures in a bargaining game with incomplete information. Game-theoretic Models of Bargaining. Cambridge: Cambridge University Press, 1985. 99-114 [17] Kreps D M, Wilson R. Sequential equilibria. Econometrica, 1982, 50(4): 863-894 [18] 王飞跃. 计算实验方法与复杂系统行为分析和决策评估. 系统仿真学报, 2004, 16(5): 893-897Wang Fei-Yue. Computational experiments for behavior analysis and decision evaluation of complex systems. Journal of System Simulation, 2004, 16(5): 893-897 [19] 王飞跃, 邱晓刚, 曾大军, 曹志冬, 樊宗臣. 基于平行系统的非常规突发事件计算实验平台研究. 复杂系统与复杂性科学, 2010, 7(4): 1-10Wang Fei-Yue, Qiu Xiao-Gang, Zeng Da-Jun, Cao Zhi-Dong, Fan Zong-Chen. A computational experimental platform for emergency response based on parallel systems. Complex Systems and Complexity Science, 2010, 7(4): 1- 10 [20] 王飞跃, 蒋正华, 戴汝为. 人口问题与人工社会方法: 人工人口系统的设想与应用. 复杂系统与复杂性科学, 2005, 2(1): 1-9Wang Fei-Yue, Jiang Zheng-Hua, Dai Ru-Wei. Population studies and artificial societies: a discussion of artificial population systems and their applications. Complex Systems and Complexity Science, 2005, 2(1): 1-9 [21] 焦李成, 刘静, 钟伟才. 协同进化计算与多智能体系统. 北京: 科学出版社, 2006.Jiao Li-Cheng, Liu Jing, Zhong Wei-Cai. Coevoutionary Computation and Multi-agent Systems. Beijing: Science Press, 2006. [22] 王飞跃. 人工社会、计算实验、平行系统——关于复杂社会经济系统计算研究的讨论. 复杂系统与复杂性科学, 2004, 1(4): 25-35Wang Fei-Yue. Artificial societies, computational experiments, and parallel systems: a discussion on computational theory of complex social-economic systems. Complex Systems and Complexity Science, 2004, 1(4): 25-35 [23] 王飞跃, 王晓, 袁勇, 王涛, 林懿伦. 社会计算与计算社会: 智慧社会的基础与必然. 科学通报, 2015, 60(5-6): 460-469Wang Fei-Yue, Wang Xiao, Yuan Yong, Wang Tao, Lin Yi-Lun. Social computing and computational societies: the foundation and consequence of smart societies. Chinese Science Bulletin, 2015, 60(5-6): 460-469 -




 下载:
下载: